In classical mechanics we can describe the state of a system by a set of two numbers {\(\vec{R}, \vec{p}\)} where \(\vec{R}\) is the position of the object and \(\vec{p}\) is its momentum. The law of dynamics (given by Newton's second law, \(\sum{\vec{F}}=m\frac{d^2\vec{R}}{dt^2}\)) describes how the state of the object changes with time. The law of dynamics is deterministic. This means that if you know the initial state {\(\vec{R}_0,\vec{p}_0\)} of the system you can use the law of dynamics to fully determine the future state {\(\vec{R}(t),\vec{p}(t)\)} of the system at any future time \(t\).
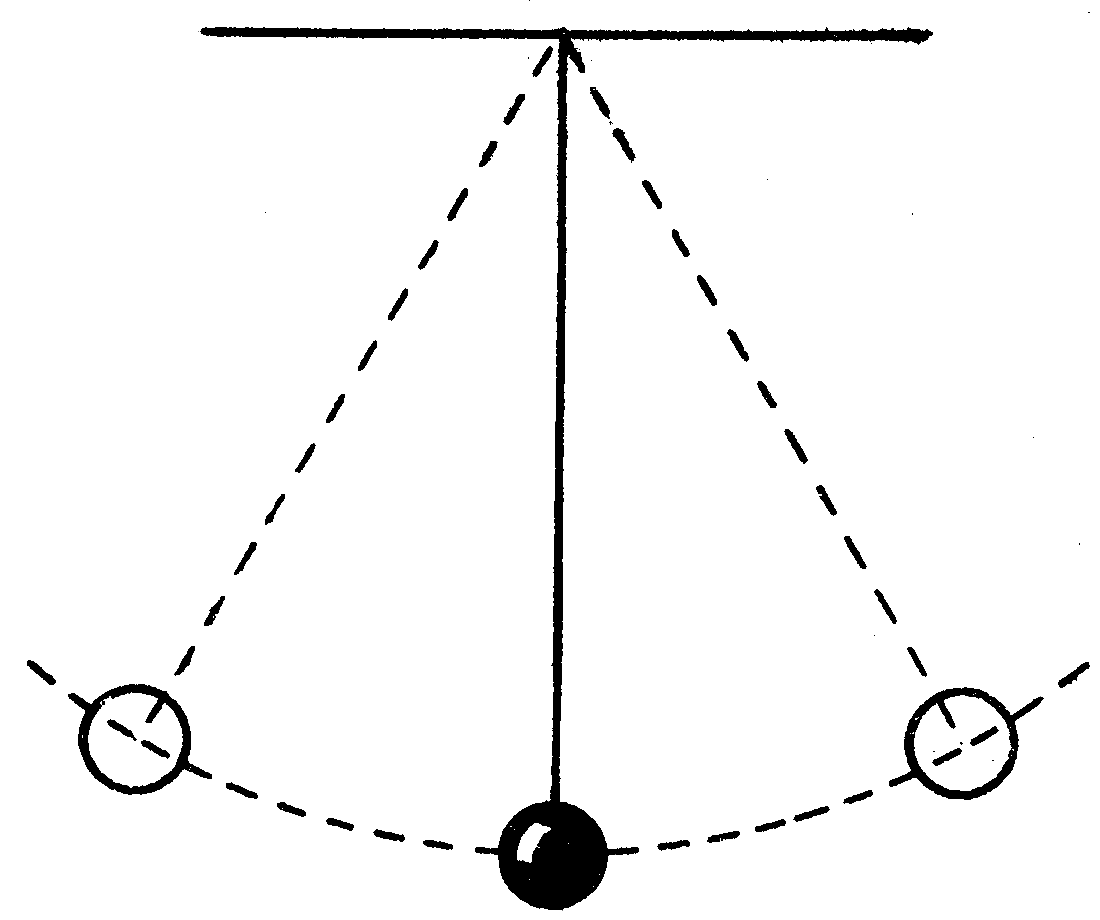
Figure 1: The motion of a simple pendulum is an example of the time-reversibility of Newton's second law. Given the initial state of the pendulum, Newton's second law can be used to determine the past or future state of the system. For example, one could determine the velocity and position of the pendulum at any past or future time given its present state.
The law of dynamics is reversible. This means that if you took two identical systems which start out in different initial states (say {\(\vec{R}_1(t_0),\vec{p}_1(t_0)\)} and {\(\vec{R}_2(t_0),\vec{p}_2(t_0)\)} respectively), they will evolve with time according to the law of dynamics in such a way that they remain in different states. Suppose however the states {\(\vec{R}_1(t_0),\vec{p}_1(t_0)\)} and {\(\vec{R}_2(t_0),\vec{p}_2(t_0)\)} evolved into the same state {\(\vec{R}(t),\vec{p}(t)\)}. If the only information you had was {\(\vec{R}(t),\vec{p}(t)\)} then, using the law of dynamics, there would be no way to know for sure whether this state evolved from {\(\vec{R}_1(t_0),\vec{p}_1(t_0)\) or {\(\vec{R}_2(t_0),\vec{p}_2(t_0)\)}. Because Newton’s law of dynamics is reversible this will not happen and the two systems will stay in different states (say {\(\vec{R}_1(t),\vec{p}_1(t)\)} and {\(\vec{R}_2(t),\vec{p}_2(t)\)}) for all times \(t\). A consequence of this is that if you know the state of either system at time \(t\) you can always use the law of dynamics to determine the state of either system at an earlier time \(t_0\).
In quantum dynamics we assume that the states of different isolated systems are deterministic and reversible. (Do not confuse the states being deterministic with the measurements being deterministic—the latter, of course, is not deterministic.) If two different systems start out in two different states \(|\psi(t_0)⟩\) and \(|\phi(t_0)⟩\) then they will remain in different states and \(|\psi(t)⟩\) and \(|\phi(t)⟩\) will stay different for all times \(t\). A consequence of this is that the inner product \(⟨\psi(t)|\phi(t)⟩\) will remain unchanged.
Figure 2: Schrodinger's equation is deterministic because the past or future quantum state \(|\psi(t)⟩\) of a quantum system can be determined with infinite precision. But, in general, a quantum system can be in a superposition of many different states where the measurement of any physical quantity is uncertain. For example, if anything the size of a small molecule or smaller passes through a double-slit in a double-slit experiment, where it hits the screen (which is to say, its final position) is uncertain (see illustration above). The quantum state of a quantum system is deterministic; but the eigenvalue that you'll measure after an experiment is done is the quantity which is not deterministic in quantum mechanics. Source: http://abyss.uoregon.edu/~js/21st_century_science/lectures/lec13.html
Scrodinger's time-dependent equation is the single most important equation in quantum mechanics. It is used to determine how any state \(|\psi(t)⟩\) of a quantum system changes with time; at all times \(t\), you'll know what \(|\psi(t)⟩\) is. This equation is also used to determine the probability \(P(L,t)\) of measuring any physical quantity \(L\) at any time \(t\). What the two functions \(|\psi(t)⟩\) and \(P(L,t)\) are depends on the total energy of the system (which is associated with the Energy operator \(\hat{E}\)) and the initial state \(|\psi(0)⟩\) of the system. All you need are these two initial conditions to determine the entire future of the system. In classical mechanics the state of a particle is specified by two numbers—the position \(\vec{R}\) and the momentum \(\vec{p}\) of the particle. Newton's second law—\(\sum{\vec{F}}=m\frac{d^2\vec{R}}{dt^2}\)—can be used to determine the entire future of the state {\(\vec{R}, \vec{p}\)} of the system. The state {\(\vec{R}, \vec{p}\)} encapsulates everything about the system: if you know what \(\vec{R}\) and \(\vec{p}\) are, then you can determine the kinetic and potential energy \(KE\) and \(PE\), the angular momentum \(\vec{L}\), and every other physical quantity associated with the system. According to classical mechanics if we knew the state {\(\vec{R}_i, \vec{p}_i\)} of every particle in the universe, we could in principle use Newton's second law \(\sum{\vec{F}}=m\frac{d^2\vec{R}}{dt^2}\) to determine the entire future of the universe. We could determine the state {\(\vec{R}, \vec{p}\)} of every particle and know every measurable quantity associated with each particle at any time \(t\). We would know everything.
In quantum mechanics if we knew the initial state \(|\psi_i(0)⟩\) of every particle in the universe, we could use the quantum analogue of Newton's second law—namely Schrodinger's time-dependent equation—to determine the future state \(|\psi_i(t)⟩\) of every particle in the universe. But where quantum mechanics differs from classical mechanics is that the state \(|\psi_i(t)⟩\) does not encapsulate everything about the system—rather it encapsulates everything that can be known about the system, which isn't everything. Each particle would have its own wavefunction \(\psi_{i,j}(t)\); in general there would be a probability amplitude associated with any physical measurement. Although the probability function \(P(L,t)\) is deterministic, the measurement of any physical quantity \(L\) is inherently probabilistic. Therefore there is an inherent randomness built into the cosmos.
This article is licensed under a CC BY-NC-SA 4.0 license.