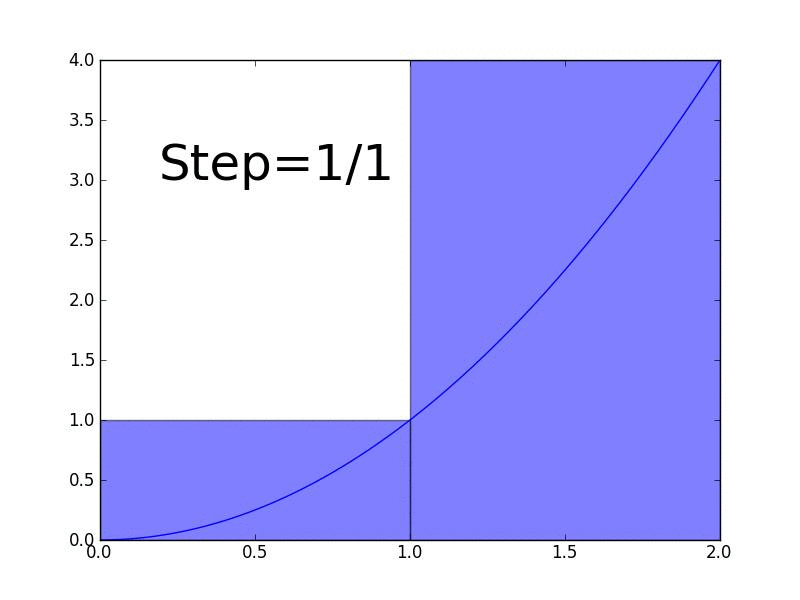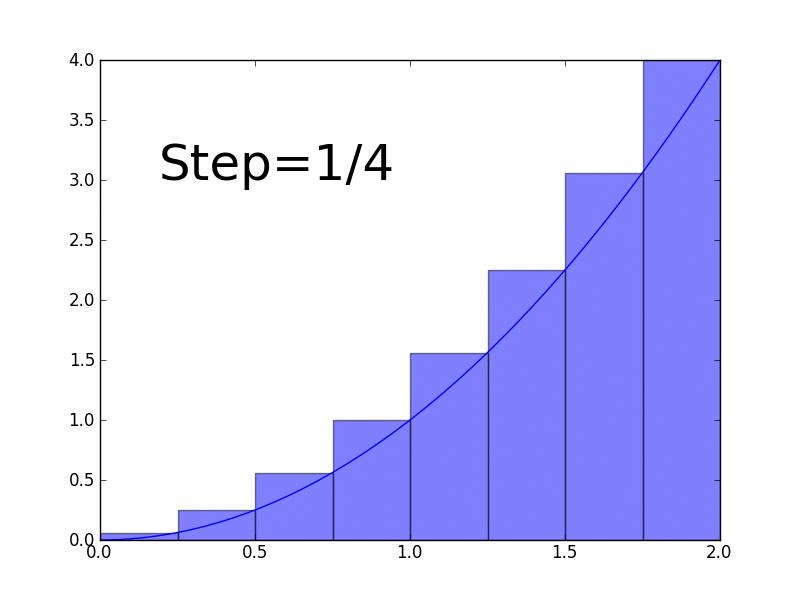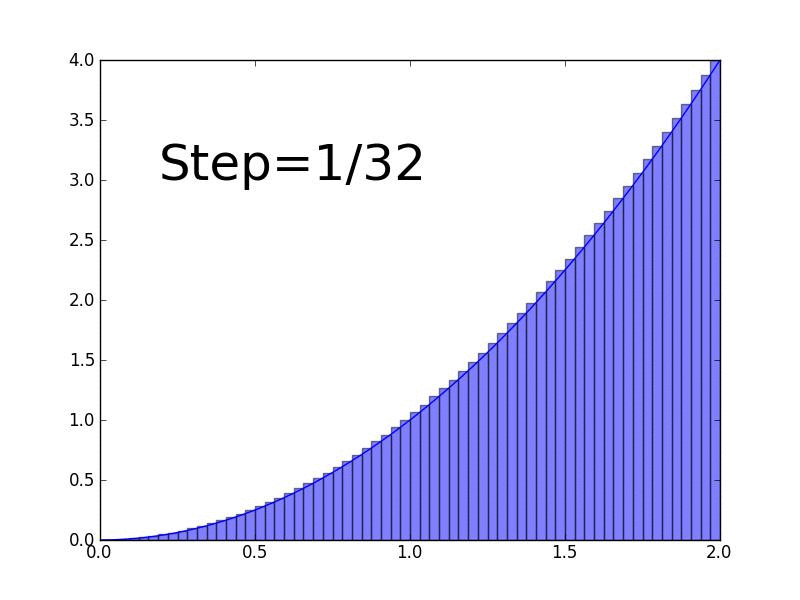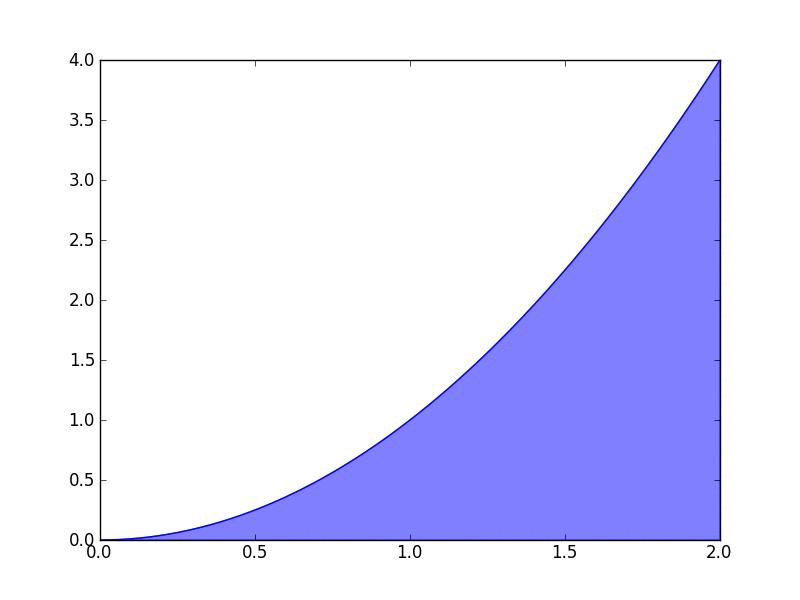
In this lesson, we define an integral as the Riemann sum as the number of rectangles approaches infinity.

In this lesson, we'll find the integral of any arbitrary function \(kx^n\) where \(k\) and \(n\) are any finite numbers such that \(n≠-1\).

In this lesson, we'll use the concept of a definite integral to calculate the arc length of a curve.

Using Newton's law of gravity and the concept of the definite integral, we can find the total gravitational force exerted by a rod on a particle a horizontal distance \(d\) away from the rod.

To find the gravitational force exerted by a disk on a particle a height \(h\) above the center of the disk, we must use Newton's law of gravity and the concept of a definite integral.

To find the gravitational force exerted by a sphere on a particle of mass \(M\) outside of that sphere, we must first subdivide that sphere into many very skinny shells and find the gravitational force exerted by anyone of those shells on \(m\). We'll see, however, that finding the gravitational force exerted by such a shell is in of itself a somewhat tedious exercise. In the end, we'll see that the gravitational force exerted by a sphere of mass \(M\) on a particle of mass \(m\) outside of the sphere (where \(D\) is the center-to-center separation distance between the sphere and particle) is completely identical to the gravitational force exerted by a particle of mass \(M\) on the mass \(m\) such that \(D\) is their separation distance.

In previous lessons, we learned that by taking the integral of some function \(f(x)\) we can find the area underneath that curve by summing the areas of infinitely many, infinitesimally skinny rectangles. In this lesson, we'll use the concept of a double integral to find the volume underneath any smooth and continuous surface \(f(x,y)\) by summing the volumes of infinitely many, infinitesimally skinny columns.

Line integrals are used to find the area between a surface \(f(x,y)\) and any arbitrary curve. In this lesson, we'll define the idea of line integral and derive a formula for calculating them.

In the previous lesson, we defined the concept of a line integral and derived a formula for calculating them. We learned that line integrals give the volume between a surface \(f(x,y)\) and a curve \(C\). In this lesson, we'll learn about some of the applications of line integral for finding the volumes of solids and calculating work. In particular, we'll use the concept of line integrals to calculate the volume of a cylinder, the work done by a proton on another proton moving in the presence of its electric field, and the work done by gravity on a swinging pendulum.

For a vector field \(\vec{F}(x,y)\) defined at each point \((x,y)\) within the region \(R\) and along the continuous, smooth, closed, piece-wise curve \(c\) such that \(R\) is the region enclosed by \(c\), we shall derive a formula (known as Green’s Theorem) which will allow us to calculate the line integral of \(\vec{F}(x,y)\) over the curve \(c\).