Law of reflection
Suppose that a light ray travels along the straight line \(QO\) until it strikes a surface at the point \(O\) as
illustrated in Figure 1. If the light ray strikes the surface at an angle \(θ_i\) relative to the line which is perpendicular to the surface, then that light ray might get reflected off of the surface depending on what kind of material the surface is made of. If the light ray does in fact get reflected—and by that, I mean "bounce" off the surface—then it'll be reflected at an angle \(θ_f\) (which is also measured relative to the perpendicular) and travel along some different path that we'll call \(OP\). It is an empirical fact that \(θ_i=θ_f\); this empirical fact is known as the law of reflection. This has been a well-known, observable fact for a very long time—since the first century in fact.
Figure 1: A light ray traveling along the line \(QO\) comes into contact with a surface at an \(θ_i\) (where \(θ_i\) is measured relative to the line perpendicular to the surface). Once this light ray comes into contact with this surface, it is reflected at an angle \(θ_r\) (\(θ_r\) is also measured relative to the perpendicular). According to the law of reflection, \(θ_i=θ_r\). Image credit: Download for free at http://cnx.org/contents/60b4727b-829e-4ea7-9238-9140b6a1b20c@4.
Snell's law: the law of refraction
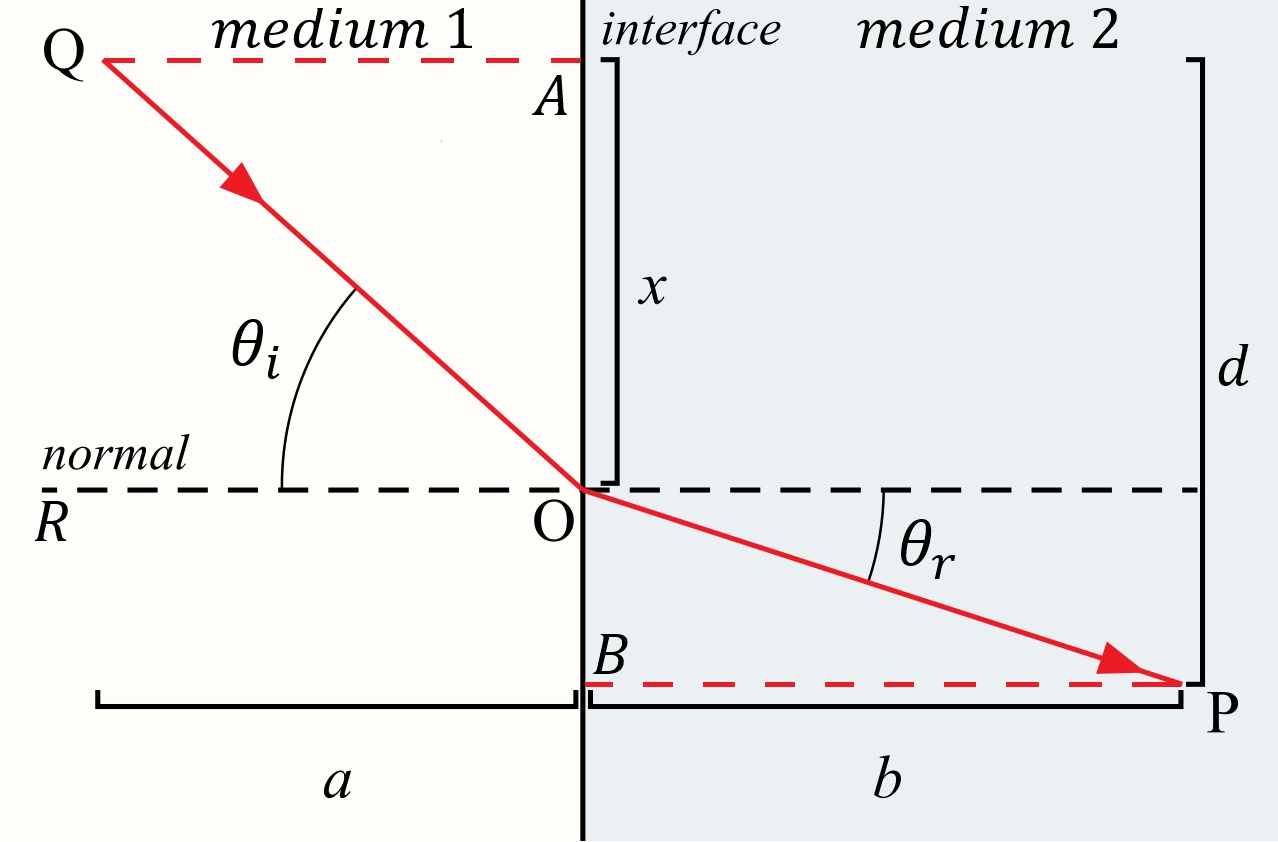
But, suppose that the path \(QO\) through which the light ray traversed is a different medium than that of the surface and below the surface. (I have labeled these different media medium 1 and medium 2 in Figure 2.) For example, suppose that medium 1 in Figure 2 is air and medium 2 is water. Then, because the two media are different, instead of the light ray reflecting off of the surface it might instead get refracted through the surface. Refraction is just a technical term that phycisists use to describe a light ray passing through one medium and then passing through another medium. If \(θ_i\) is the angle that the light ray makes with the line \(RO\) when it reaches the surface (this angle is known as the angle of incidence), and if \(θ_r\) is the angle between the light rays new path \(OP\) (the path it takes as it travels through medium 2) and the line \(RO\) (a line which is perpendicular to the surface), then \(θ_i≠θ_r\). The reason why \(θ_i≠θ_r\) is because when the light ray enters into a different medium, its path—although still a straight line—gets "bent." The mathematical relationship between \(θ_i\) and \(θ_f\), and how the angle \(θ_f\) could be determined given that the angle \(θ_i\) is known, remained a mystery for over a millennium.
Figure 2: As a light ray travels along the line \(QO\) through medium 1 and comes into contact with the interface between medium 1 and medium 2, it gets refracted—meaning, it passes through medium 2. Image credit: By Smedlib (Own work) [CC BY-SA 4.0 (https://creativecommons.org/licenses/by-sa/4.0)], via Wikimedia Commons
But during the seventeenth century, a mathematician named Pierre de Fermat cracked this mystery. It was known then that, provided the medium doesn't change, then light will travel at a constant velocity (meaning that both the speed of alight ray and the direction of a light ray is traveling in will not change) through that medium. It was also known that the speed of light can be different\(^1\) in different media. When the astronomer Christian Hyugans discovered that light can act like a wave, he used this fact (the fact that the speed of light is different in different media) to explain what physically causes the path of a light ray to get "bent" as it travels from one medium into another. But we'll explain that in more detail in a separate lesson.) With this fact in mind, we'll let \(v_1\) denote the speed of the light ray in medium 1 and we'll also let \(v_2\) denote the speed of the light ray in medium 2. Fermat also had to make a postulation (an "educated guess"): namely, if \(P\) and \(Q\) denote the initial and final locationas of a light ray, respectively, then the light ray will take the path which will allow it to get form \(P\) to \(Q\) in the least amount of time possible. This axiom is known as the principle of least time. This principle explains why light travels in a straight line if the medium that it goes through doesn't change: because that's the path which allows the light ray to travel form one place to another place in a period of time that is shorter than if it took any other path. This principle also explains the law of reflection. The reason why after the light ray gets reflected off of the surface (see Figure 1) it takes a path \(OP\) such that \(θ_f=θ_i\) is because that is the path which allows the light ray to get ot its final location in the least time.
But this principle can also be used to explain why a light ray traveling from one medium to another takes the path \(QOP\) (see Figure 2) where \(θ_i=θ_f\). Fermat used his principle of least time and the techniques of calculus for finding minima and maxima to explain refraction. Since, according to the principle of least time, the light ray will take a path between points \(Q\) and \(P\) which minimizes the time \(t\) it travels, the problem that Fermat faced was to find the path which minimizes \(t\). To be able to use the language of single-variable calculus (see lesson, Finding the Minima and Maxima of a Function) to find the minimum of some quantity, we have to express that quantity as a function of a single independent variable. Thus we need to somehow express \(t\) as a function of some variable. Let's say that the point \(O\) in Figure 2 can be any point on the surface. This means that the two line segments \(QOP\) represents any path that the light ray could take to get from \(Q\) to \(P\). Let's also say that \(x\) is the horizontal distance between the point \(O\) and the line \(QA\) in Figure 2. Since \(O\) can be any point on the surface, \(x\) is clearly a variable. Let's also represent the height of \(Q\) above the \(x\)-axis by \(a\) and the height of \(P\) below the \(x\)-axis by \(b\). Lastly, we'll let \(d\) be the vertical distance (distance along the \(x\)-axis in Figure 2) between \(Q\) and \(P\); a consequence of this is that the length of the line \(OB\) is \(d-x\). With all of the relevant quantities stated, using the Pythagorean theorem and the fact that the speed of light is a constant in each medium, we can express the time \(t\) for the light ray to go from \(P\) to \(Q\) as
$$t=\frac{\sqrt{a^2+x^2}}{v_1}+\frac{\sqrt{(d-x)^2+b^2}}{v_2}.\tag{1}$$
Notice that since \(a\), \(b\), \(d\), \(v_1\) and \(v_2\) are all constants, we have accomplished our goal of expressing \(t\) as a function of a single variable and we can now apply the techniques of calculus that we learned in previous lessons to find the minimum of \(t\). Taking the derivative of both sides of Equation (1) with respect to \(x\), we have
$$t'(x)=\frac{1}{v_1}(\frac{1}{2})\frac{1}{\sqrt{a^2+x^2}}(2x)+\frac{1}{v_2}(\frac{1}{2})\frac{1}{\sqrt{(d-x)^2+b^2}}(2)(d-x)(-1).\tag{2}$$
Equation (2) simplifies to
$$t'(x)=\frac{x}{v_1\sqrt{a^2+x^2}}-\frac{d-x}{v_2\sqrt{(d-x)^2+b^2}}.\tag{3}$$
If we set \(t'(x)=0\) in Equation (3), then Equation (3) will become
$$\frac{x}{v_1\sqrt{a^2+x^2}}-\frac{d-x}{v_2\sqrt{(d-x)^2+b^2}}=0.\tag{4}$$
As you can see from Figure 2,
$$sinθ_i=\frac{x}{\sqrt{a^2+x^2}}$$
and
$$sinθ_r=\frac{d-x}{\sqrt{(d-x)^2+b^2}}.$$
Substituting \(sinθ_i\) and \(sinθ_r\) into Equation (4), we have
$$\frac{sinθ_i}{v_1}-\frac{sinθ_r}{v_r}=0,$$
or
$$\frac{sinθ_i}{sinθ_r}=\frac{v_1}{v_2}.\tag{5}$$
Equation (5) is known as Snell's law (or the law of refraction) and it gives us the relationship between \(θ_i\) and \(θ_r\) for various different kinds of materials.
This article is licensed under a CC BY-NC-SA 4.0 license.
References
1. Kline, Morris. Calculus: an Intuitive and Physical Approach. Dover, 1998.
2. OpenStax, The Law of Reflection. OpenStax CNX. Jul 5, 2012 http://cnx.org/contents/60b4727b-829e-4ea7-9238-9140b6a1b20c@4
3. Smedlib. “Snells law Diagram B vector.” Wikipedia, Smedlib, Wikipedia, Jul 3, 2017, https://commons.wikimedia.org/wiki/User:Smedlib.
Further Reading
1. Refraction and light bending. Retrieved from https://www.khanacademy.org/science/in-in-class-12th-physics-india/in-in-ray-optics-and-optical-instruments/in-in-reflection-and-refraction/a/refraction-and-light-bending.
2. Snell's law. (2017, October 26). In Wikipedia, The Free Encyclopedia. Retrieved 15:13, October 31, 2017, from https://en.wikipedia.org/w/index.php?title=Snell%27s_law&oldid=807213926
Notes
1. Actually, the speed of a light is a universal constant. Technically speaking, it is the average speed of light which can change from one medium to another.